The theory of spontaneous quantum gravity: an overview

SCEST21: Schrodinger's Cat, and Einstein's Space-time, in the 21st Century

A blogspot for discussing the connection between quantum foundations and quantum gravity

Managed by: Tejinder Pal Singh, Physicist, Tata Institute of Fundamental Research, Mumbai

If you are a professional researcher / student researching on these topics, and would like to post an article here with you as author, you are welcome to do so. Please e-mail your write-up to tpsingh@tifr.res.in and it will be uploaded here.


Keywords: Quantum foundations; Quantum gravity; Schrodinger's cat; Spontaneous collapse theory; 
Trace dynamics; Non-commutative geometry; Spontaneous quantum gravity; Classical general relativity 
***************************

December 5, 2019

The theory of Spontaneous Quantum Gravity: an overview

YouTube Video   https://youtu.be/lJk_mE8K8uw

Tejinder Singh

As we have seen in the previous posts, we would like an underlying theory for spontaneous collapse, which also provides  a relativistic description of collapse. Trace dynamics goes a good part of the way, by deriving quantum field theory as the equilibrium statistical mechanics of an underlying matrix dynamics with global unitary invariance. Brownian motion fluctuations about equilibrium can provide the origin of spontaneous localisation, subject to a few assumptions. Trace dynamics operates at the Planck scale, but assumes space-time to be flat Minkowski space-time. Quantum theory is then derived by coarse graining trace dynamics over time scales much larger than Planck time. Quantum dynamics is thus a low energy emergent phenomenon, emerging after this coarse graining. 

It is desirable though that we include gravity in trace dynamics, considering that Planck scale physics is involved. We also recall our other goal to have a formulation of quantum (field) theory without classical space-time. It turns out that the TD formalism can help us do that, if we can find a way to incorporate gravity. This would then also be a theory of quantum gravity. We also emphasised earlier that quantum gravity must dynamically explain the absence of space-time superpositions in the classical limit. That goal will be achieved here, because TD already has a mechanism (fluctuations) to explain spontaneous collapse.

So, how do we bring in gravity? It cannot be brought in simply as classical general relativity. That is not allowed by the Einstein hole argument: the matter in TD is not classical, whereas classical matter fields are needed to give operational meaning to the point structure of classical space-time. We also recall that trace dynamics describes matter degrees of freedom as matrices, which do not commute with each other. We search for a similar matrix/operator type description of gravity, which should not already be tied to quantisation of classical gravity. Because quantum theory should in fact emerge from the sought for [trace dynamics + gravity] after coarse-graining over Planck time scales. Thus we seek a matrix dynamics description of [matter + gravity], on the Planck scale.

Fortunately, such a matrix type description of gravity exists - it is the non-commutative geometry (NCG) program discovered and developed  by Alain Connes and collaborators. From our point of view, keeping trace dynamics in mind, NCG could be introduced as follows: what kind of geometry would we get if we raised space-time points (described by real numbers) to the status of matrices/operators? Recall we did just this kind of thing for material particles and gauge fields in TD. Similarly, our space-time points now become operators, which in general do not commute with each other. 

In a mathematical approach, this can be understood as follows. Physical space, or space-time, is described by the laws of geometry. It can be mapped to an algebra, by assigning coordinates to the points of space. Then geometric properties (such as curvature) can be described in terms of functions on the algebra. This of course is a commutative algebra - real numbers commute with each other. After mapping the geometry of the space to a (commutative) algebra, we now take the following step: we make the algebra non-commutative. This is precisely what is achieved by elevating points of the space (or space-time) to matrices. The matrices do not commute with each other, and hence we have a non-commutative algebra. Now we ask: what kind of geometry such a non-commutative algebra describe?! That `geometry’, we call non-commutative geometry. There is no corresponding geometry in the sense in which we relate geometry to space, but we can talk of analogous concepts: e.g. what is the curvature of a non-commutative space? 

One immediately notices the striking parallel between trace dynamics on the one hand, and NCG on the other. Both obtain by elevating classical point structures to the status of non-commuting operators. The former arrives at a matrix dynamics for matter and gauge fields. The latter arrives at a matrix description of geometry. Now classical general relativity couples classical matter to Riemannian geometry: matter curves space-time. We then expect matter described by trace dynamics to couple to non-commutative geometry: matrix matter `curves’ non-commutative space-time. Thus we intend to built a matrix theory of matter + gravity by unifying trace dynamics with non-commutative geometry. And we demand that this matrix dynamics have the following properties: when we perform the statistical mechanics of this gravity-based matrix dynamics, we should obtain a quantum theory of gravity at equilibrium. Fluctuations should become important for macroscopic systems (macroscopic to be defined) and spontaneous collapse should then come into play, giving rise to an emergent classical space-time and classical matter fields, such that the classical space-time has a Riemannian geometry which obeys the laws of general relativity. Fortunately, a mathematical formalism for such a programme has been developed: this is the theory of Spontaneous Quantum Gravity (SQG). SQG is in fact trace dynamics + trace gravity (i.e. NCG). From here, quantum gravity, quantum (field) theory, and classical general relativity, and classical dynamics, are all emergent phenomena.

What would such a matrix gravity look like, mathematically? Naively, we might want to make coordinates into operators, raise each metric component to an operator, try to construct a curvature tensor operator, and somehow couple it to the trace Lagrangian for matter fields in TD. But this does not work, for various reasons. It is technically difficult to make an invariant four-volume from the determinant of the metric, when each metric component is an operator. It all does not have the right feel, to say the least. Furthermore, classical space-time has been lost; how will we even describe time evolution and hence the dynamics, in matrix gravity? 

Fortunately, the formalism of NCG shows the way forward. One can properly describe concepts such as distance and metric, in a non-commutative geometry. What is very important is that NCG seems to provide a new fundamental time parameter - a property unique to non-commutative geometry, not found in commutative algebras. We will return to this in some detail in future posts - for now we just accept and employ this time parameter, which we will call Connes time. We lost space-time, but we recover time, and that is adequate for dynamics. Time is more fundamental than space.

When we raise space-time points to operators/matrices, like in TD, we can try to use the TD language of Grassmann matrices. In classical GR, metric is a field that lives on space-time. That won’t do now: trying to make something live on an operator. We expect the space-time operator to describe space-time geometry itself, and indeed that does happen. Also, we need to ask - the Grassmann matrix that represents space-time geometry: should it be just bosonic, or should it have a fermionic part too? I don’t at present know he answer to this important question. For now, we work with a Grassmann even (i.e. bosonic) matrix to describe spacetime geometry. 

Since we want matrix gravity to yield GR (with matter sources) in the classical limit, we will have to specify a Lagrangian - both for gravity and for matter. Again, NCG shows the way, for gravity. There is a remarkable result in geometry, which relates curvature in a Riemannian geometry, to the Dirac operator on this space-time. Consider a Riemannian space - having a Euclidean signature. For now, and in this SQG program, we work with the Euclidean case. The Lorentzian case remains to be developed. Given a curved Riemannian space, one can write the standard Dirac operator D_B on it [`gamma-mu del-mu’] in terms of the gamma-matrices and the spatial derivatives. A result from geometry states that [expressed for now as a simplified statement] the trace of the square of the Dirac operator is equal to the Einstein-Hilbert action [`integral of R root(g)’]. Isn’t that surprising - that the sum of the eigenvalues of the Dirac operator on a space is connected to Riemannian curvature on that space. The eigenvalues of the Dirac operator are connected to gravity - in fact they *are* gravity, as we will see later. The metric can be connected to these eigenvalues. 

So we have this operator, D_B² on a Riemannian space. We can make the algebra of coordinates non-commutative, and we will still have this square of the Dirac operator: it describes curvature on a non-commutative space. And we nave the Connes time, labelled say tau, to describe evolution. We can now make contact with trace dynamics; recall that the trace Lagrangian is trace of an operator polynomial in configuration variables and their velocities. The trace polynomial coming from NCG is trace of square of the Dirac operator. Remembering that in quantum mechanics the Dirac operator is like momentum, we now introduce in our theory a bosonic operator/matrix q_B such that its derivative with respect to Connes time is the Dirac operator D_B. This is the defining condition for q_B while the defining condition for the Dirac operator is as before: it becomes the ordinary Dirac operator on a Riemannian space, and there it relates to the Ricci scalar and the Einstein-Hilbert action. In matrix gravity, the action describing gravity is the (Connes) time integral of the trace of the squared Dirac operator. This has just the form expected from trace dynamics. Moreover, the Lagrange equation resulting from this action is also very simple: the momentum is constant in time, and the configuration variable evolves linearly with time.

Next, we must include matter, because we after all want to derive spontaneous localisation of matter, from the SQG theory. At this stage in this programme we consider matter fermions only, leaving the consideration of (bosonic) gauge fields and non-gravitational interactions for later. So we have to have a way to include say Dirac fermions, in the language of trace dynamics. One thing we can anticipate is that these will be described by fermionic Grassmann matrices. But what should the Lagrangian be, keeping also in mind that we also have the Dirac operator at hand. We could construct a trace Lagrangian for every fermion in the theory, add up these Lagrangians, and add this to the trace Lagrangian for gravity (described above), integrate it over Connes time, and that could give the action for matrix dynamics. 

However, we do not go on that path, for conceptual reasons. Let us ask the question: what is the gravitational  effect of an electron? An electron, being quantum mechanical, is all over space; so why must we distinguish the gravitational effect of the electron from the electron itself? This situation is unlike that of a classical object, say planet earth, where the object is localised in space, and its gravitational field is spread out everywhere. So we propose to introduce the concept of an `atom’ of space-time-matter [STM] which is a combined description of the fermionic part (say the electron) to be described by a fermionic operator q_F, and its gravitation part, to  be described by the bosonic q_B. Thus, we define the operator q for an STM atom, written in terms of its bosonic and fermionic parts: q = q_B + q_F. For instance, in matrix gravity, an electron along with its gravity is an STM atom - it comes with its own operator space-time coordinates, its own Dirac operator. Further, we define the fermionic part of the Dirac operator, D_F to be the Connes time derivative of q_F. And the full Dirac operator D by D = D_B + D_F. Recall that the original Dirac operator D_B is bosonic. The Lagrangian for an STM atom is the trace of the square of D, and the action is the time integral of this Lagrangian. There is one such term for each STM atom. We can write the total action for matrix gravity simply as: 

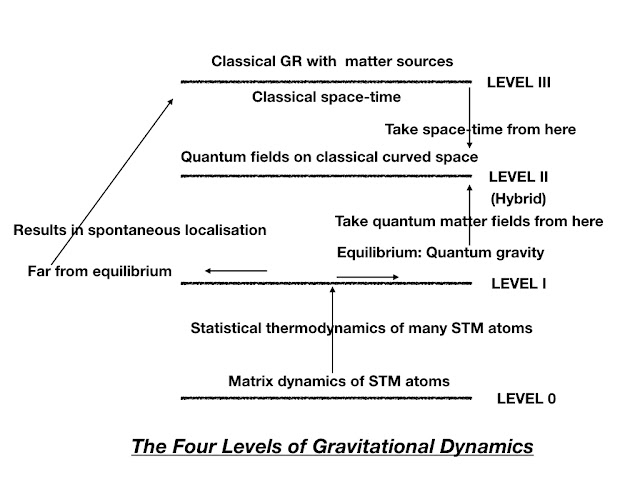
This is nice. From here we can derive quantum gravity, quantum theory, classical general relativity, all as emergent phenomena. To begin with, one easily obtains the Lagrange equations for the bosonic and fermionic part of each STM atom. The momenta are constant, and the configuration variables evolve linearly with time. Because of unitary invariance, there again is a conserved Adler-Millard charge. The following diagram helps us understand where to go next.  

What we have described so far takes place at Level 0. There are only two fundamental constants at Level 0 - Planck length and Planck time. And there is the conserved Adler-Millard charge. Every STM atom has only one associated parameter, a length scale L, which eventually gets interpreted as Compton wavelength (L can be different for every atom). At Level 0,  there is no Planck’s constant, no Newton’s gravitational constant, no concept of mass nor spin: all these are emergent at higher levels. At level 0, there is only the length scale L, from which mass and spin emerge subsequently at Level I. How do the STM atoms interact with each other? `Collisions’ and entanglement are possible mechanisms - this aspect is currently under investigation.

Level 0 is a Hilbert space on which the operators describing STM atoms live, and evolve in Connes time. Dynamics take place at the Planck scale, as in trace dynamics. There is no space-time here. Space-time and the laws of general relativity emerge at Level III, as a consequence of spontaneous localisation. We can say that space-time arises as a consequence of collapse of the wave-function; more specifically, the part of the wave function that describes the fermions. The bosonic part does not undergo localisation, and becomes space-time and its curvature.

Like in trace dynamics, we would like to know what the emergent dynamics at low energies is, if we average Level 0 dynamics over time scales much larger than Planck time. For this we perform the statistical thermodynamics of the STM atoms described by the action given in the equation above. What emerges, at equilibrium at Level I, are the standard quantum commutation relations, and Heisenberg equations of motion, separately for the bosonic and fermionic parts of each STM atom. Evolution is still in Connes time. Planck’s constant emerges too, and hence Newton’s gravitational constant can be defined, using Planck’s constant along with Planck time and Planck length. The mass of an STM atom is defined in terms of its length L, which length hence can be interpreted as its Compton wavelength. The Schwarzschild radius of an STM atom is defined as square of Planck length divided by L. One can transform to the Schrodinger picture dynamics as well, and define quantum entanglement. Thus what we have at equilibrium at Level I is a quantum theory of gravity, emergent from the Level 0 matrix gravity dynamics. If we would like to know what is the gravitation of an electron, we can answer that question at Level I, or at Level 0, but not at Level II or III. Note that this Level I quantum gravity is a low energy phenomenon! It does not have anything to do with the Planck scale, but rather comes into play whenever a background space-time is not available. This Level I quantum gravity is also the sought after description of quantum (field) theory without classical time.

If a sufficiently large number of STM atoms get entangled, something very interesting takes place. If the total mass of the entangled system of STM atoms goes above Planck mass, the effective Compton wavelength of the full system goes below Planck length. The approximation that we can coarse grain the Level 0 dynamics over times larger than  Planck times breaks down. This is what we mean by fluctuations becoming important. The entangled system experiences rapid Planck scale fluctuations, an anti-self-adjoint part from the fermionic trace Hamiltonian is no longer negligible, and the entangled system undergoes extremely rapid spontaneous localisation. The localisation of the fermionic parts of many such entangled systems gives rise to the macroscopic bodies of the universe. Their bosonic parts together describe classical gravity, which is shown to obey the laws of classical general relativity. 

Those STM atoms which do not undergo spontaneous localisation are to be described at Level 0 or Level I. Or, if we neglect their gravity, we can describe them at the hybrid Level II, after borrowing the space-time part from Level III. This is how we  conventionally do quantum (field) theory.

The theory of Spontaneous Quantum Gravity makes the following predictions:

1. Spontaneous localisation (the GRW theory) is a prediction of this  theory, and the GRW theory is being tested in labs currently. If the GRW theory is ruled out by experiments, this proposal will be ruled out too.

2. SQG predicts the novel phenomena of quantum interference in time, and spontaneous collapse in time. 

3.The theory predicts the Karolyhazy length as a minimum length. This is testable and falsifiable.

4. This theory predicts that dark energy is a quantum gravitational phenomenon.

5. The theory provides an explanation for black hole entropy, from the microstates of STM atoms.

In forthcoming posts, we will discuss the SQG theory as well as its predictions in some detail. SQG is a candidate cover theory for general relativity, in the sense discussed in the first post. It explains the emergence of the classical world from quantum gravity, without having to resort to any interpretation of quantum mechanics.

The origin of spontaneous localisation: Trace Dynamics III

SCEST21: Schrodinger's Cat, and Einstein's Space-time, in the 21st Century

A blogspot for discussing the connection between quantum foundations and quantum gravity

Managed by: Tejinder Pal Singh, Physicist, Tata Institute of Fundamental Research, Mumbai

If you are a professional researcher / student researching on these topics, and would like to post an article here with you as author, you are welcome to do so. Please e-mail your write-up to tpsingh@tifr.res.in and it will be uploaded here.


Keywords: Quantum foundations; Quantum gravity; Schrodinger's cat; Spontaneous collapse theory; Trace Dynamics; Statistical Mechanics
_____________________________________________
December 3, 2019

The origin of spontaneous localisation: Trace Dynamics III

Tejinder Singh

We have seen that the theory of trace dynamics gives rise to relativistic quantum (field) theory as an emergent phenomenon, after one constructs the equilibrium statistical thermodynamics of the underlying theory. This emergence opens up the possibility that spontaneous localisation is also a consequence of trace dynamics, in the following sense. When one arrives at the thermodynamic approximation by constructing the equilibrium configuration by applying statistical mechanics to the underlying microscopic theory, it is assumed that statistical  fluctuations away from equilibrium are negligible. Under certain circumstances though, fluctuations could become important. A situation of precisely this kind gives rise to spontaneous localisation, starting from the underlying trace dynamics, subject to certain assumptions. These assumptions will be justified when we incorporate gravity into trace dynamics (to be discussed in subsequent posts). 

Recall that the Adler-Millard charge is anti-self-adjoint, whereas the trace Hamiltonian is assumed to be self-adjoint.  We wish to consider the impact of statistical fluctuations on the emergent quantum dynamics. The description of these  fluctuations, and the stochastic correction that they imply to the trace Hamiltonian, is controlled by the Adler-Millard charge.  While the averaged Adler-Millard charge is equipartitioned, and proportional to Planck’s constant times a unit imaginary matrix, the fluctuations need not be equipartitioned. Moreover, one can by hand assume that the fluctuations can have an imaginary part, thus resulting in the Hamiltonian having an anti-self-adjoint part. [In the theory of spontaneous quantum gravity, this does not have to be put in by hand - the fundamental Hamiltonian at the Planck scale naturally has an anti-self-adjoint part]. 

The inclusion of imaginary fluctuations around equilibrium paves the way for spontaneous localisation to arise, provided some additional assumptions are made. It is assumed that such localisation takes place only for fermions (i.e. only in the matter sector, not for bosonic fields). Furthermore, only the non-relativistic case is considered, I.e. the Schrodinger equation for matter particles. Stochastic linear terms are added to the Hamiltonian, to represent fluctuations about equilibrium. Since the fluctuations include an imaginary part, the evolution of the modified Schrodinger equation does not preserve the norm of the state vector. [Norm preservation is essential, if one is to derive the Born probability rule]. The requirement of norm preservation is sought to be justified on empirical grounds, because particle number is conserved in the non-relativistic theory, and is related to the norm. Hence, a new state vector - which preserves norm - is defined by scaling the old state vector by its  norm. This makes the modified Schrodinger equation into a stochastic non-linear differential equation, which with the further assumption of no superluminal signalling, acquires precisely the form as in the GRWP theory of spontaneous collapse. And collapse does take place at a faster rate when more and more particles are entangled with each other. Entanglement enhances spontaneous collapse, making the equilibrium unstable. In fact, we have to recall that the mean dynamics arose after averaging over time scales larger than Planck time. Precisely this assumption - that such an averaging can be done - breaks down for macroscopic systems, as we will see in spontaneous quantum gravity! Moreover, there we will also find justification for the assumptions made above.

The take away note from this post is that trace dynamics contains within itself the roots for explaining spontaneous collapse, because quantum dynamics in the first place arises as a statistical thermodynamics approximation to the underlying matrix dynamics. There is every cause for investigating circumstances where fluctuations become important. And the classical world arises precisely because of such circumstances. Trace dynamics is presently the only known theory which provides a theoretical basis for spontaneous collapse.

A major limitation of trace dynamics is that while it operates at the Planck scale, it assumes space-time to  be Minkowski space-time. This however is clearly only a `transitory’ assumption, meant to be eventually relaxed. Until recently, it was not clear how to incorporate gravity as a matrix dynamics. We now know that Alain Connes’ non-commutative geometry programme enables us to do that. In so doing, we will also see how various assumptions made in trace dynamics get justified. 

Incorporating gravity into trace dynamics finally helps us understand that spontaneous localisation arises because the fundamental Hamiltonian at  the Planck scale is not self-adjoint. It does not have to be. Only the emergent Hamiltonian in quantum theory has to be self-adjoint. We will also see how we arrive at a formulation of quantum theory which does not depend on classical space-time: this was one of our stated goals. We will also have a theory of quantum gravity which dynamically explains absence of superposition of classical space-time geometries. The theory also explains the origin of black hole entropy, and suggests a quantum gravitational origin for dark energy.

In the next post, we provide an overview of spontaneous quantum gravity, and in subsequent posts we discuss the theory in some detail.

The origin of spontaneous localisation: Trace Dynamics II

SCEST21: Schrodinger's Cat, and Einstein's Space-time, in the 21st Century

A blogspot for discussing the connection between quantum foundations and quantum gravity

Managed by: Tejinder Pal Singh, Physicist, Tata Institute of Fundamental Research, Mumbai

If you are a professional researcher / student researching on these topics, and would like to post an article here with you as author, you are welcome to do so. Please e-mail your write-up to tpsingh@tifr.res.in and it will be uploaded here.


Keywords: Quantum foundations; Quantum gravity; Schrodinger's cat; Spontaneous collapse theory; Trace Dynamics; Statistical Mechanics

____________________________________________________

December 2, 2019

The origin of spontaneous localisation: Trace Dynamics II

Tejinder Singh

As we saw earlier, trace dynamics is a matrix dynamics with a global unitary invariance, which operates at the Planck scale. Now we ask this question: suppose we do not wish to examine the dynamics on time scales of the order of Planck time or even smaller times, and we coarse-grain the trace dynamics over time steps much larger than Planck times, what is this time-averaged dynamics? It is shown, using the techniques of statistical mechanics, that this averaged dynamics is quantum (field) theory. No specific fine-tuning is done in the underlying trace dynamics so as to ensure that the emergent dynamics is quantum dynamics. Of central importance is the fact that as a  consequence of the global unitary invariance of the trace Lagrangian, trace dynamics possesses a conserved charge, the Adler-Millard charge. This charge has dimensions of action, and its equipartition as a result of doing the statistical mechanics is what is responsible for the emergence of quantum theory. It is plausible to assume that since the emergent theory results from averaging over time intervals much larger than Planck time, the energy scale associated with the emergent quantum system is much smaller than Planck energy. And this is of course true for the systems we currently study in laboratories while applying quantum field theory to the standard model of particle physics. It is already implicit in this analysis above that the dynamical laws on the Planck scale are not those of quantum field theory, but those of trace dynamics. This proposal will become much more plausible when we incorporate gravity into trace dynamics, in the theory of Spontaneous Quantum Gravity.

The principles of statistical mechanics are generally employed to derive the properties and thermodynamic laws for macroscopic systems, starting from the laws of atomic theory. Central to these principles is the fact that a macroscopic system is made of an enormously many constituent particles, whose individual motions we know how to describe, but we are not interested in. The macro-state is then determined by maximising the entropy made from all the microstates consistent with the values of physical attributes describing the macro-state (say constant temperature, or constant energy).

In the present instance of trace dynamics, these principles of statistical mechanics are being put to a different use, not for finding what happens to trace dynamics when we are considering a macroscopic system. Instead, we want to know what is the average dynamics of a system of one or more matrices obeying trace dynamics, when the dynamics is coarse-grained over time scales larger than Planck time scale. To find this average dynamics, we consider an ensemble of a very large number of matrices, each of which obeys trace dynamics at the Planck scale. Each one of them follows a different trajectory in the phase space, but we can assume [the ergodic hypothesis] that the ensemble average of the dynamics at any one time represents the long time average (i.e. the averaged dynamics of a matrix when coarse-grained over intervals larger than Planck time). We want to know the equilibrium ensemble, subject to the constancy of the trace Hamiltonian and the Adler-Millard charge.

One starts by defining a volume measure in the phase space made from the matrix elements - if there are N matrices in the trace dynamics, there is one canonical pair in the phase space for each of the elements of every one of the N matrices. A Liouville theorem is proved, namely that trace dynamics evolution preserves a volume measure in phase space. Next, the equilibrium density  distribution function in phase space is defined, which as usual gives the probability of finding the system in a given infinitesimal phase space volume. It is also shown that the ensemble average of the AM charge is a constant times a unit matrix, and since this constant has dimensions of action, it will eventually be identified with Planck’s constant subsequent to the emergence of the quantum dynamics.

The Boltzmann entropy is defined from the density distribution, it being determined by the number of matrix microstates consistent with a given constant value of the trace Hamiltonian and of the Adler-Millard charge. The equilibrium distribution is obtained by maximising the entropy subject to the constancy of these two conserved quantities. Next, we must recall that the energy scale we are interested in is much smaller than Planck scale; as a consequence the properties of the equilibrium distribution are determined by the AM charge, not by the trace Hamiltonian. A set of so-called Ward identities are proved for the equilibrium distribution, these being a generalised analog of the energy equipartition theorem in statistical mechanics. Important consequences follow from these identities. The AM charge is equipartitioned over all the bosonic and fermionic degrees of freedom and the equipartitioned value is identified with Planck’s constant. Recalling that the AM charge was defined in terms of the fundamental commutators and anti-commutators, it follows that the q, p degrees of freedom, when averaged over the equilibrium canonical ensemble, obey the commutation relations of quantum theory. Lastly, it is shown that the canonically averaged configuration variables and momenta obey the Heisenberg equations of motion of quantum theory. This last result follows because the (canonical averages of the) functions which define the time derivatives in the (first-order) Hamilton’s equations of trace dynamics get related to the canonically averaged commutators which eventually appear in the Heisenberg equations of motion. 

In this sense, quantum theory is an emergent phenomenon. When the equations of trace dynamics are coarse-grained over time intervals larger than Planck time, the emergent dynamics is quantum dynamics. This comes about because of the existence of the non-trivial Adler-Millard charge, unique to trace dynamics. The contact with quantum field theory is made by defining the Wightman functions of quantum field theory in terms of the emergent canonical averages of corresponding degrees of freedom in trace dynamics. One arrives at the standard relativistic quantum field theory for bosons and fermions. Since the Heisenberg equations of motion are now available, an equivalent Schrodinger picture dynamics can also be formulated.

Trace dynamics is one approach to derive quantum theory from symmetry principles, rather than arriving at quantum theory through the ad hoc recipe of `quantise the classical theory’. How can one justify the necessity of statistical mechanics in arriving at quantum theory? We recall that there is also an aspect of randomness/probabilities related to quantum mechanics - this being the aspect that comes into play during a quantum measurement. Randomness and probabilities are characteristic of statistical mechanics, specifically when fluctuations away from equilibrium become important. It is these fluctuations which are responsible for spontaneous localisation. We will take up this novel aspect of trace dynamics in the next post.

The origin of spontaneous localisation: Trace Dynamics I

SCEST21: Schrodinger's Cat, and Einstein's Space-time, in the 21st Century

A blogspot for discussing the connection between quantum foundations and quantum gravity

Managed by: Tejinder Pal Singh, Physicist, Tata Institute of Fundamental Research, Mumbai

If you are a professional researcher / student researching on these topics, and would like to post an article here with you as author, you are welcome to do so. Please e-mail your write-up to tpsingh@tifr.res.in and it will be uploaded here.


Keywords: Quantum foundations; Quantum gravity; Schrodinger's cat; Spontaneous collapse theory; Trace Dynamics; Statistical Mechanics; Matrix Models

____________________________________________________

December 1, 2019

The origin of spontaneous localisation: Trace Dynamics I

Tejinder Singh

We have seen earlier that spontaneous localisation is a falsifiable but ad hoc modification of the Schrodinger equation, for explaining the absence of macroscopic position superpositions. One would like to derive this collapse theory from an underlying dynamics, based on a symmetry principle. One such theory, known as Trace Dynamics (TD) has been developed by Stephen Adler (IAS, Princeton) and collaborators. The best source to read about this theory is Adler’s book `Quantum theory as an emergent phenomenon’ [Cambridge University Press, 2004].

Put in brief, Trace Dynamics is the dynamics of matrix models which obey a global unitary invariance. Quantum (field) theory is emergent as the statistical mechanics of these matrix models. TD is assumed to operate at the Planck scale, although space-time is assumed to be Minkowski space-time. The matrix models describe the dynamics of fermionic matter, as well as of gauge fields, although no specific form of the Lagrangian of the theory is prescribed. Gravity is not included.

What is the motivation behind trace dynamics? One would not like to arrive at quantum theory by quantising a classical theory. This is considered unsatisfactory because the classical theory is only a limiting case of quantum theory - one should not have to know the limit of a theory to construct the theory; it should be the other way around. [We do not construct special / general relativity by `relativising’ Newtonian mechanics / gravitation. The relativity theories are built from their own symmetry principles, and yield the Newtonian theories in the limit]. Trace dynamics is a first principles theory, from which quantum, and classical mechanics, are emergent as approximations.

In TD, particles and fields are not described by real numbers, but by matrices (equivalently operators). Consider for instance the Newtonian Lagrangian dynamics of a collection of particles with configuration variables q_i . From these variables and their time derivatives we can make the Lagrangian, and from there obtain the equations of motion. To construct trace dynamics, assume instead that each of the q_i is a matrix/operator. We will construct its corresponding velocity by taking the time derivative of the matrix, which is equivalent to taking time derivative of each matrix element. Given this set of configuration matrices and their velocities, the Lagrangian of TD is constructed by making a polynomial from matrix products, and then taking the matrix trace of this polynomial. Thus the Lagrangian is a scalar (a real number), rightfully called the trace Lagrangian. The action in TD is the time integral of this trace Lagrangian. Lagrange equations of motion are obtained by extremising the action by varying it with respect to the operator configuration variables. This requires the introduction of a `trace derivative’ - which is a natural method for differentiating  trace of a polynomial w.r.t. an operator. The resulting Lagrange equations are operator equations. Also, TD is constructed to be a Lorentz invariant theory. Importantly, the configuration variables and their conjugate momenta, all non-commute with each other. Unlike in quantum theory, the commutators in TD are arbitrary and time-dependent, in general.

What, one might ask, is the point of these matrices/operators? What is the physical interpretation of their matrix elements? TD is a pre-quantum theory, more general than quantum theory, operating at the Planck scale; a theory from which quantum mechanics will be derived as an emergent approximation, at energy scales below the Planck scale. The eigenvalues and eigenstates of these matrices represent possible values that these degrees of freedom can take, and superposition  is possible too; yet the rules of evolution are not those of quantum theory.

Trace dynamics can be extended to fields too, by dividing three-space into cells and assigning one q-operator for every cell, which then represents the field value in that cell. Alternatively, one can take the continuum limit of the N-particle trace dynamics. 

The elements of the matrices in TD are made from complex numbers and complex Grassmann numbers. A complex Grassmann number is made from two real Grassmann numbers. A real Grassmann number is made from products of Grassmann elements. Grassmann elements anti-commute with each other, unlike ordinary numbers which commute with each other. Thus the square of a Grassmann number is zero. A product of an even number of Grassmann elements commutes with every Grassmann element, and together with unity these form an even-grade Grassmann algebra, which is used to represent bosonic fields. A product of an odd number of Grassmann elements anti -commutes with other odd number products of Grassmann elements: together these form the odd-grade sector of the Grassmann algebra. These are used to represent fermionic fields. A general matrix made from complex Grassmann numbers can be written as a sum of two matrices, one made from even-grade elements (and called bosonic) and one made from odd-grade (and called fermionic).

In matrix dynamics the trace Hamiltonian of the system is conserved, as can be expected. In addition though, trace dynamics possesses a fascinating conserved charge, which results from the global unitary invariance of the trace Lagrangian and trace Hamiltonian. This charge is known as the Adler-Millard charge, it has no analog in ordinary classical dynamics, and is responsible for the emergence of quantum theory from trace dynamics. It is given by the sum (over bosonic degrees of freedom) of the commutators [q,p], minus the sum (over fermionic degrees of freedom) of the anti-commutators {q,p}. Note that this charge has the dimensions of action. If the Hamiltonian  is self-adjoint, the AM charge is anti-self-adjoint. If the Hamiltonian were to also have an anti-self-adjoiint piece, the AM charge picks up a self-adjoint component. One can also define a generalised Poisson bracket in trace dynamics, and express the dynamics as Hamilton’s equations of motion, and also in terms of Poisson brackets. In general, evolution in trace dynamics is not unitary, and differs from the evolution given by the Heisenberg equations of motion in quantum theory.

Trace dynamics in many ways resembles matrix models that have been studied in quantum field theory. However, in matrix models, canonical quantum commutation relations are imposed on the matrix elements, thus leading to the standard quantisation. Unlike matrix models though, one does not quantise a trace dynamics. Instead one asks, what does the coarse grained dynamics look like, if trace dynamics is averaged over time-intervals much longer than Planck time, and one is interested in the emergent dynamics at energy scales much smaller than Planck scale? It turns out that this emergent dynamics is quantum dynamics. This is established by applying the conventional techniques of statistical mechanics to trace dynamics, a topic which we will take up in the next post.